Geodesy in Hungary and the Relation to IAG
around the turn of 19th/20th Century-A Historical Review
By József Ádám (Technical University of Budapest)
Introduction
Geodesy has a long tradition in Hungary. Due to the special connection of Hungary to former Austria in 19th century, beside Prussia and Saxony, Hungary as part of the former Austria is a founder member country of the Mitteleuropäische Gradmessung in 1862, predecessor of the International Association of Geodesy (IAG). Survey control networks, namely the primary triangulation and levelling networks in Hungarian part were also established by the Military Geographic Institute in Vienna (that is, k.u.k. Militärgeographisches Institut) until 1919, see e.g.Sterneck (1908).
In the field of geodetic science, separate research and scientific activities were carried out at the Universities in Budapest. These activities can be connected with Professors István Kruspér, Lajos Bodola and Károly Oltay at the Department of Geodesy, Technical University and to Professor Loránd Eötvös at the Department of Physics, Budapest University. They were all members of the Hungarian Academy of Sciences.
István Kruspér (1818-1905) wrote the first book of geodesy in Hungarian issued in 1869 (1st edition) and in 1885 (2nd edition). He actively participated in the works of the Commission Internationale du Métre (CIM) and he acted as chairman of one of the Subcommission of CIM. He was Professor of Geodesy and Head of the Department between 1850-1894.
Lajos Bodola (1859-1936) was successor of István Kruspér at the Technical University as Professor of Geodesy and Head of the Department between 1894-1913. He also actively participated in the works of the CIM respectively Comité International des Poids et Mesures (CIPM). Bodola was elected to be Secretary General of the CIMP in 1922. He was the first national representative of Hungary to the Internationale Erdmessung (from 1897) and convenor of the XVth General Assembly of the Internationale Erdmessung in Budapest in September 20-28, 1906. He made some high precision geodetic measurements for research works of Loránd Eötvös.
Károly Oltay(1881-1955) was successor of Lajos Bodola at the Technical University as Professor of Geodesy and Head of the Department between 1913-1955. By invitation and on behalf of Loránd Eötvös, Oltay made extensive astronomical and gravitational (pendulum) measurements in different parts of Hungary, see Oltay (1910).
Loránd Eötvös(1848-1919) was born in the year of the Hungarian revolution and fight for independence. His father József Eötvös as a writer and politician of great renown, was elected minister of religion and education in the first independent government of Hungary after the revolution. (In recognition of his literary work, József Eötvös became an associate member of the Academy in 1835, a full member in 1839 and in 1866 he was elected president.) Loránd Eötvös graduated at Heidelberg University where he studied physics, mathematics and chemistry. After graduation Eötvös returned home in 1871, and successfully applied for the post of lecturer, advertised by the faculty of theoretical physics at the Pest University. This university now bears his name, as Loránd Eötvös University. After a short period of lecturing, in 1872 he was publicly honoured by the king, who awarded him the chair of theoretical physics. In 1874 he was allowed to give lectures in experimental physics and four years later he became professor in this field. He was then given the task of uniting the departments of experimental and theoretical physics, and was appointed as director to the newly established Institute of Physics. In 1873 he became associate member of the Academy, a full member in 1883 and in 1889 he was elected president. Amongst his offices he became minister of religion and education for seven months in 1894.
In addition to activities as a scientific researcher and lecturer, Eötvös played an important role in organizational work, thus promoting the development of the natural sciences in Hungary. He received awards at home and abroad for his scientific achivements and works. Among the very many awards he received the French Legion of Honour, the Franz Josef award from the Hungarian king, the Saint Sava award from the king of Serbia. He was also elected honorary member of the Prussian Royal Academy of Sciences in Berlin and was given honorary doctorates from Jagello University in Cracow and the Norwegian Royal Frederick University in Christiania (now Oslo). In appreciation of his scientific achivements, some important rules and objects are linked with the name of Eötvös (Fig.l) in geodesy, namely Eötvös-effect, Eötvös-correction, Eötvös Torsion Balance, Eötvös Unit, Eötvös-tensor, see Groten (1980), Hotine (1969), Marussi (1985), Moritz and Hofmann-Wellenhof (1993) and Torge (1991).
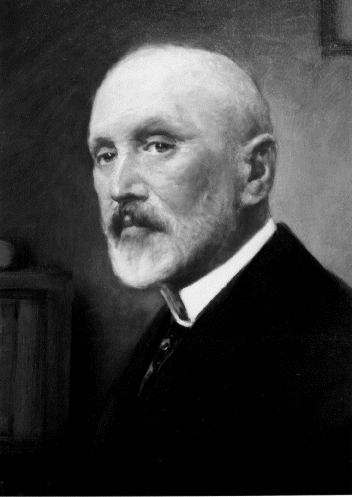
Figure 1
. Baron Loránd Eötvös as painted by Gyula Éder in 1913 (courtesy of Eötvös Loránd Geophysical InstituteIn the next paragraph the activities of Loránd Eötvös related to geodesy are summarized shortly. The Eötvös Loránd Geophysical Institute (ELGI) of Hungary celebrated his founder with a permanent exhibition, where beside the personal and family relics all original Eötvös torsion balances and other instruments are exhibited. Eötvös Virtual Museum is available on the WWW at http://www.elgi.hu/museum/index.htm
Geodesy related activities of Loránd Eötvös
Loránd Eötvös started to investigate the gravitation and magnetism in 1886. From this date until his death, he was concerned with these two fields (Selényi, 1953; Szabó, 1992, 1998 and 1999). In his research on the spatial changes in gravitation, he used a modified version of Coulombs balance. His research method was based on two fundamentals:
| the strict theoretical physical aspect of the process, and | |
| the construction of an unbelievably sensitive instrument. |
Eötvös built two different types of torsion balance for carrying out his gravitational investigations. The first type was a light horizontal beam suspended on a torsion wire with platinum masses attached to each end so that the masses were at the same level (curvature variometer). This type was identical in form with the instrument used by Cavendish. The curvature variometer measures values, which give the deviation of equipotential surfaces of gravity from spherical shape, and give the directions of minimum curvature. The second type has a platinum mass attached to one end of a horizontal beam, while on the other end a platinum cylinder hangs on a wire so that this cylindrical mass is at a lower level than the mass at the other end (horizontal variometer). In both cases the beam turns around the torsion wire in a horizontalplane and is deflected from the torsion-free position of the wire by the horizontal components of the forces of gravity. This seemingly insignificant modification was Eötvös most important invention, in fact this second version is known as the Eötvös Torsion Balance. Principle of the Eötvös' torsion balance is given in Torge (1991).
The horizontal variometer gives the rate of change of gravity over a horizontal distance of one centimetre. The horizontal variometer also furnishes the curvature values if the instrument is set up in at least five azimuths. The unit of gradient and curvature are named after Eötvös as Eötvös Unit(EU). 1 EU = 10-6 mGal/cm; that is, if the horizontal gradient is 1 EU, the gravity acceleration at two neighbouring points 1 cm apart difference 10-12 g. The gradients, that is second partial derivatives of the gravity potential W constitute the elements of Eötvös tensor (or gravity gradient tensor). The mechanical parts of the instruments were designed and built in 1890 under the guidance of Eötvös in cooperation with N. Suess in Suess Precision Tool Workshop, predecessor of the Hungarian Optical Works (MOM). Calibration of the instruments were carried out in Eötvös Laboratory and the first instrument was completed in 1891. In the same year the first observations were carried out. A modified version of the horizontal variometer specially designed for fieldwork, was completed in 1898 (Fig.2.a.). It was shown and awarded a prize at the Expo of Paris in 1900. In order to increase the efficiency of fieldwork, Eötvös constructed a double instrument with two balances in antiparallel arrangementin1902 (Fig.2.b.).
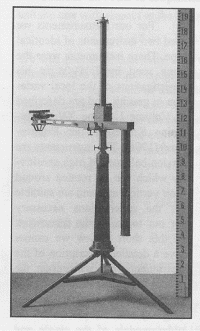
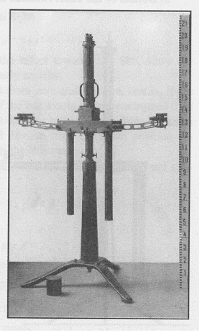
Figure
2 Single and double torsion balance (Eötvös, 1908)(a) Designed for field work in 1898. This was an award winner at the World Exhibition in Paris in 1900. b) Designed in 1902. Eötvös and his colleagues used this instrument in their experiments to study the equivalence of inertial and gravitationalAt the beginning Eötvös experimented with his instruments in the laboratory of the university. He carried out his first field measurements on Ság hill in Transdanubia in 1891 (Fig.3), where he proved that errors had been made in the relative pendulum measurements carried out by Sterneck; Sterneck was an Austrian geodetic surveyor who had carried out measurements in the same area seven years earlier. Eötvös first report on gravitation was written in 1888 for the Academy. In 1896 he published his fundamental paper (Eötvös, 1896), in which he gave a theoretical and practical summary of his experiments to date.

Figure
3. This photo documents the first torsion balance field measurements carried out by Eötvös on Ság hill of Transdanubia in August 1891. Eötvös can be seen at the telescope, in front of him is Lajos Bodola, next to him sitting on the ground is Radó Kövesligethy, while the standing person is Károly Tangl. In later years all of them were to become eminent professors (courtesy of Eötvös Loránd Geophysical Institute)The first experiments on a larger area using the Eötvös' balance took place in the winter of 1901 on the frozen surface of Lake Balaton. Eötvös chose the mirror-like frozen surface of the lake to carry out his measurements so that he would not have to concern himself with the disturbing effect of topographic masses. He continued his survey work in the winter of 1903, completing measurements at altogether 40 different stations. From the results of his torsion balance survey it was established that parallel to the axis of the lake ran a tectonic line. This was the first geological conclusion based on torsion balance measurements. As a results of his success when he presented his results in Paris in 1900,Eötvös gravitational experiments became the focus of international attention. The high degree of sensitivity of his instrument was doubted by some. And it was not until the XVth Congress of the Internationale Erdmessung held in Budapest in 1906 at which he spoke about his latest experiments that Eötvös claims received general recognition (Eötvös, 1908). He also made it possible for interested foreign scientists to observe his torsion balance measurements carried out in the field - in the Arad region. The participants of the conference found Eötvös' research so significant that they (namely G.H. Darwin and F.R. Helmert) petitioned the Hungarian government requesting that increased financial help be given for gravitational research. The Hungarian government agreed to the suggestion and from 1907 onwards a separate fund was allocated for Eötvös' gravitational studies. From this time onwards geophysical research was recognized in Hungary as a separate field of science in its own right.
At first Eötvös' gravitational measurements were carried out for geodetical purposes, but from the very beginning Eötvös had wondered what geological conclusions could be deduced from the results of his work. At the XVIth and XVIIth Congress of the Internationale Erdmessung Eötvös wrote his reports on the practical application of the torsion balance (Eötvös, 1910 and 1913). In 1916 on the initiative of Hugo Böckh, an eminent Hungarian geologist, torsion balance measurements were carried out in the region of Egbell (now Gbely, Slovakia) where oil was produced from a recognized anticlinal structure. The aim of these measurements was to establish the extent to which the effect of the oil-bearing anticline is reflected in the results of torsion balance measurements. On the basis of the measurements carried out at 92 points the contours of the anticlinal oil field were clearly ascertained. These results, which proved the efficiency of the torsion balance in oil prospecting, heralded the birth of petroleum geophysics and at the same time paved the way towards world renown for Eötvös and his balance. In the 1920s and 1930s many oil fields were discovered throughout the world with the help of Eötvös' ingenious instrument. Note that the latest development of gravity gradiometry is outlined by Bell (1998). The gradiometry is currently developed for use in an artificial earth satellite. Hopefully, the satellite gradiometry mission called GOCE will be a reality soon (Rummel, 1986 and Rummel, 1997).
Towards the end of the 19th century, the central question of geodesy was that of determining the geoid, i.e. the equipotential surface of gravity determining the shape of the Earth. From torsion balance observations the difference between the two main curvatures of the equipotential surface - i.e. its deviation from spherical - passing through the centre of mass of the balance can be computed.
The first regional surveys called attention to the phenomenon that the equipotential surface, and with it the shape of the Earth, can deviate from the regular even within small distances. Eötvös, from his Arad survey, prepared the first ever detailed level surface (geoid) contour map, in 1906 (Fig.4). At the same time, the question of projecting surface points to sea level was discussed: it was proposed that instead of the generally accepted means of using the normal of the ellipsoid, a plumb-line should be used for the projection. The basic condition of this method is knowledge of the deflection of the vertical, which - for a long time - could only be determined in detail by the torsion balance.

Figure
4 Vertical deflections and a level surface contour map in the region of Arad. Contour interval of the level surface undulations (referred to Bessel-ellipsoid isolines: 2cm.Although best known for his torsion balance, Eötvös also developed a gravimeter. (In this innovative work he was ahead of his time by about two decades.) It was completed in 1901, and was based on the bifilar principle.
However, the experimental measurements carried out with this instrument failed to meet his expectations, so he did not publish his activities in this field. Nevertheless, his gravimeter still exists today (see Eötvös Virtual Museum on WWW), an example of the depth of his love for experimentation. Note that gravimeters were far less sensitive than torsion balances but what they loss in sensitivity they gained in portability. They were far less cumbersome and gave far more rapid results so they gradually took over the role of torsion balances in mineral exploration in the 1940s.
Eötvös became concerned with the question of the proportionality of the inertial and gravitational mass by the end of the 1880s. In order to examine this phenomenon, he used his sensitive torsion balance (Fig.2.b). He examined the state of equilibrium of the balance by attaching masses of different composition to each end of the arm of the balance. If the magnitude of gravity depended on the composition of the mass, the state of equilibrium should change in each case. This phenomenon did not occur. In 1908 Eötvös and his colleagues, (Jenő Fekete and Dezső Pekár), perfected their measurements to such an extent that they were able to establish that the difference between the inertial and gravitational mass was at most 1/200,000,000 (Fig.5). Their paper on the subject won them the Benecke award of Göttingen University in 1909. The experiments carried out by Eötvös and his colleagues on the proportionality of inertial and gravitational mass provided fundamental support for Einstein's theory of relativity.

Figure
5. The equivalence of inertial and gravitational mass shown experimentally by Eötvös and others [Soffel, 1997]In the last years of his life, Eötvös carried out experiments, which showed that the weight of moving bodies on the Earth's surface depended on their direction and velocity. A clear explanation of this change can be given on the basis of Galilean and Newtonian mechanics. The gravitational force of the Earth is the resultant of two forces: the principal one caused by the attraction according to Newton's law, the second one the centrifugal force caused by the Earth's rotation. Since the distribution of the masses on the Earth's surface and the velocity at which the Earth rotates are constant, the weight of objects on the Earth's surface is also constant. The situation is different, however, in the case of moving objects. As the Earth rotates from west to east, the centrifugal force on a moving object is greater if it moves towards the east than towards the west. As a result of this phenomenon the weight of a body moving eastwards will decrease, while that moving westwards will increase. This means that the weight of a man walking eastwards is less than if he is walking westwards. The difference would be two grams.
It is interesting to note the circumstances that initiated Eötvös' research on this topic. An eminent researcher (O. Hecker) at the Institute of Geodesy in Potsdam had led a team to the Atlantic Ocean in 1901 and then in 1904-1905 to the Indian and Pacific Oceans, to carry out gravity measurements on moving ships. While studying Heckers results in the published report, Eötvös noticed that no consideration had been given to the forces developed by the motion of the ship. In a letter to Hecker, Eötvös pointed out the error but Hecker at first refused to give credence to this criticism. His colleagues, however, persuaded him that Eötvös was right and so in 1908 new measurements were carried out in the Black Sea to prove this phenomenon. Observations were made in two ships, one moving towards the east and one towards the west. The results substantiated Eötvös' claim. The international scientific community recognizes this phenomenon as the Eötvös Effect. The Eötvös Effect is of special importance nowadays in the field of sea and air gravimetry, see e.g. Groten (1980), Smit (1988) and Torge (1991).
In 1915 Eötvös constructed a special instrument to demonstrate this phenomenon (that is the Eötvös Effect). The device is basically a balance with a horizontal beam, with a mass attached to each end instead of pans. The balance stands on a rotating tripod (see Eötvös Virtual Museum on WWW). When the balance is rotated the mass moving towards the west will become heavier, the one moving towards the east lighter. The balance will, therefore, be deflected from its state of equilibrium. If the balance is rotated at such a speed that the rotation period equals the period of its oscillation the impulses occurring during the rotations will cause the balance to make ever-greater oscillations.
XVth General Assembly of the "Internationale Erdmessung" in Budapest in 1906 - An Identification sought
The "Internationale Erdmessung ("Association Géodésique Internationale" in French) held its XVth General Assembly in Budapest in 1906 between September 20 and 28. A detailed minutes of the meeting is given in (Bericht, 1908). On a sightseeing tour in Budapest, a group photograph on the participants was made at the Margaret Island which was not yet published up to now. A copy of this photograph is enclosed by the courtesy of the owner, the Hungarian Museum for Science and Technology which could be of interest to you.
Based on a list of the official participants, the following nineteen countries sent delegates:

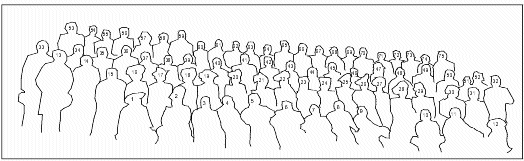 Figure 7. Identified participants on the photograph are as follows: (2) Mrs. L. Bodola, (3) T. Tasaka, (4) L. Bodola, (5) A.R.Kalmár, (6) L. Eötvös, (8) H. Kimura, (9) F.R. Helmert, (38) H. Poincaré, (39) L. Bassot, (42) G. Darboux, (43) C.E. Guillaume, (45) G.H. Darwin, (52) V. Dobrovics, (53) A. Fasching, (57) E. Weiss, (61) J.F. Hayford, (65) W. Tinter, (70) H.J. Heuvelink.
Figure 7. Identified participants on the photograph are as follows: (2) Mrs. L. Bodola, (3) T. Tasaka, (4) L. Bodola, (5) A.R.Kalmár, (6) L. Eötvös, (8) H. Kimura, (9) F.R. Helmert, (38) H. Poincaré, (39) L. Bassot, (42) G. Darboux, (43) C.E. Guillaume, (45) G.H. Darwin, (52) V. Dobrovics, (53) A. Fasching, (57) E. Weiss, (61) J.F. Hayford, (65) W. Tinter, (70) H.J. Heuvelink.
(Argentina) Professor F. Porro de Somenzi, Director of the Observatory of the La Plata University. (Austria) Professor W. Tinter, Technical University of Vienna; Vice-Admiral A. Ritter von Kalmar, Vienna; F. Lehrl from the Vienna Military Survey and Professor E. Weiss, Director of the Vienna Observatory. (Belgium) Major L. Gillis, Director of Military Mapping Institute, Brussels. (Denmark) General von Zachariae, Klampenborg. (France) General L. Bassot, President of the Internationale Erdmessung, Member of the French Academy of Sciences and Director of the Observatory, Nice; A. Bouquet de la Grye, Member of the French Academy of Sciences, Paris; R. Bourgeois, Director of the Geodetic Branch, Military Survey, Paris; G. Darboux, Permanent Secretary of the French Academy of Sciences, Paris; F. Hanusse, Director of the Hydrographic Service, French Navy, Paris ; Ch. Lallemand, Director of the French Survey Levelling Office, Paris and H. Poincaré, President of the French Academy of Sciences, Paris and Honorary Member of the Hungarian Academy of Sciences. (Germany) Professor W. Foerster, University of Berlin; Professor M.Haid, Technical University of Karlsruhe; Professor M. Schmidt, Technical University of Munich; Professor R. Schorr, Director of the Hamburg Observatory; Professor Th. Albrecht, Geodetic Institute, Potsdam; Colonel von Bertrab, Director of the Berlin Military Triangulation Office, Berlin; Professor E. Borrass, Geodetic Institute, Potsdam; Professor A. Börsch, Geodetic Institute, Potsdam; Professor O. Hecker, Geodetic Institute, Potsdam; Professor F.R. Helmert, Potsdam University and Director of the Geodetic Institute, Potsdam and Professor E. Becker, Director of Strassburg Observatory. (Great Britain) Sir G.H. Darwin, Professor at the Cambridge University. (Hungary) Professor Lajos Bodola, Technical University of Budapest and Baron Loránd Eötvös, Professor at the Budapest University. (Italy) General C. Crema, Director of Military Geographic Institute, Florence and Professor F. Guarducci, University of Bologna. (Japan) Generalmajor T. Tasaka, Tokyo and Dr. H. Kimura, Director of the Observatory at Mizusawa. (Mexico) A. Anguiano, Director of the Tacubaya Geodetic Commission and F. Valle, Director of the Tacubaya Observatory. (The Netherlands) Professor H. G. van de Sande Bakhuyzen, Secretary General of the Internationale Erdmessung, Director of the Leiden Observatory; Professor H. J. Heuvelink, Technical University of Delft and Dr. J. J. A. Muller, Director of the Triangulation Office at Sumatra, Zeist. (Norway) Professor O. Schiötz, Christiania (now Oslo) University. (Romania) General C.J. Bratiano, Director of the Institute of Military Geography, Bucharest and Colonel J. Cantea, Institute of Military Geography, Bucharest. (Russia) Colonel N. Artamonoff, Director of Military Topography, St. Petersburg and Dr. O. Backlund, Director of Pulkovo Observatory. (Spain) A. Galarza y Vidal, Director of the Geographic and Statistic Institute, Madrid and E. Mier y Miura, Member of the Academy of Sciences, Madrid. (Sweden) Professor P.G. Rosén, Member of the Swedish Academy of Sciences. (Switzerland) Professor R. Gautier, Director of Geneva Observatory. (USA) O.H. Tittmann, Superintendent of the U.S. Coast and Geodetic Survey, Washington and J. F. Hayford, Inspector of Geodesy at the U.S. Coast and Geodetic Survey, Washington.
Also invited were: Baron F. Podmaniczky; M. Déchy, Vice - Chairman of the Hungarian Geographic Society; V. Dobrovics, Director of the Hungarian Triangulation Office; A. Antalffy, Director of the Hungarian National Survey; Baron B. Harkányi, Member of the Hungarian National Geodetic and Geophysical Commission; Professor J. Farkas, Kolozsvár (today Cluj Napoca, Romania) University; J. Jankovich, Dr. M. Réthy, A. Schuller and Dr. I. Fröhlich, geodetic experts from Budapest, furthermore Professor C.E.Guillaume Nobel Prize Winner from Paris, France and Professor S. Shinjo from Kioto, Japan. The Press Attache of the Conference was Dr. A. Fasching, a leading Hungarian surveyor at that time and later Professor of Geodesy at Zagreb University.
If any would be able to identify some of the delegates, I would be most grateful.
Invitation to the IAG Scientific Assembly in Budapest in 2001
After 95 years, the International Association of Geodesy (IAG), one of the member associations of the International Union of Geodesy and Geophysics (IUGG) will hold its Scientific Assembly in the capital of Hungary, Budapest from September 2nd to 8th, 2001, in response to an invitation of the IAG Section of the Hungarian National Committee for the IUGG. The scientific symposia and associated meetings will be held at the building of the Hungarian Academy of Sciences. Pre-and post symposia programmes will be organized in order to support the discovery of Hungary's unique cultural treasures and its challenging natural environment. All scientists interested in IAG activities are cordially invited to participate in the Scientific Assembly in Hungary in 2001.
The scientific program, under the title Vistas for Geodesy in the New Millennium, will emphasize the interaction of geodesy with the other Earth Sciences and Engineering, and its growing contribution to the modelling of the System Earth. It will highlight the impact of major new satellite missions and the integration of new data sources with existing ones. The program will be open for new ideas, the planning of new programs, the integration of new people, and the creation of new flexible organisational structures. Special sessions are planned to feature Gravity Gradiometry from Eötvös to GOCE etc. A major effort will be made to attract a large number of graduate and undergraduate students to share the new vistas for geodesy.
As decided at the last General Assembly of the IAG in Birmingham in July, 1999 a special IAG Council Meeting will take in conjunction with this Scientific Assembly. The year 2000 will have an outstanding significance for the host country, Hungary, which celebrates the 1000th anniversary of its statehood as well as of the adoption of Christianity. Bordered by Austria, Croatia, Romania, Serbia and Montenegro, Slovakia, Slovenia and Ukraine, Hungary is the heart of Central Europe. Located at the crossroads of all these different cultures, Hungary provides a perfect bridge between Eastern and Western Europe. The Local Organizing Committee (LOC) would like to combine the high-level scientific and technical content of the Scientific Assembly with the cultur of Hungary and hopes to be a good host to all Assembly participants. At the very beginning of the new Millennium the Scientific Assembly hopefully will attract participants from all over the world.
Acknowledgements The author wishes to express his appreciation to Mrs. Ignác Bacsa for typing the text, to Mr. Frigyes Raum to call the author's attention for the photograph of the Hungarian Museum for Science and Technology, to Mr. Szabolcs Rózsa for scanning the photograph and figures and for editing the text, and to Mr. Zoltán Szabó for delivering the figures of the Eötvös Loránd Geophysical Institute (ELGI).
Bibliography.